Generalising in Mathematics by Philip Higgins

One of the most revealing aspects of ME625 and ‘Developing Thinking in Algebra’ (Mason et al 2012) is that algebra is the search for the general rule and that this generality can be uncovered in most cases, and with some practise it becomes achievable. I say this because I never felt that way in my O’ Level school maths, back in the 1980’s. Most education critique that I currently read says that the curriculum should now be taught differently, with creativity being foremost. Searching out that general rule is a creative challenge. I have learnt all this aged fifty, having graduated in BSc Mathematics and its Learning. The following extract is from my ME625 End-of-Module assignment in which I tried to step up another level and actually teach generalising to a student. It was not easy.
Finding generality through Invariance and Change
My maths learner was my son, twenty years old, and in second year university on a Product Design course. He is educated to A’ Level in Mathematics. It was my difficulties in trying to teach him generalising during earlier work, which he found confusing due to my lack of structure, that prompted me to shake up ‘Question 13.1.5 Invariance’.
Here is the course textbook question:
Task 13.1.5 Invariance
What is changing and what is the same about the three following expressions as a whole?
3 + 5 = 2 x 4 5 + 7 = 2 x 6 3 + 7 = 2 x 5
I adapted it and split the whole task into six small parts so that steady progress could be made without feeling overwhelmed by suddenly having to generalise. The ‘Dimensions-of-possible-variation’ task shown at the end allowed him to create his own version.
Part One – finding changes
My learner explores change using spot-the-difference. The image is Paul Morphy, a famous Victorian chess player. My idea is to encourage visual interest.

He stumbled with finding the last three differences. It seems my tiniest of ‘Paul Morphy tweeks’ was challenging to him but this encouraged focus.
Part Two – visualising the nth term
In part two my idea was to familiarise my learner with the general number ‘ n ‘ but without the trauma of having to extract it from a ‘sequence of numbers’ or a ‘story question’. It is a simple approach.
Question
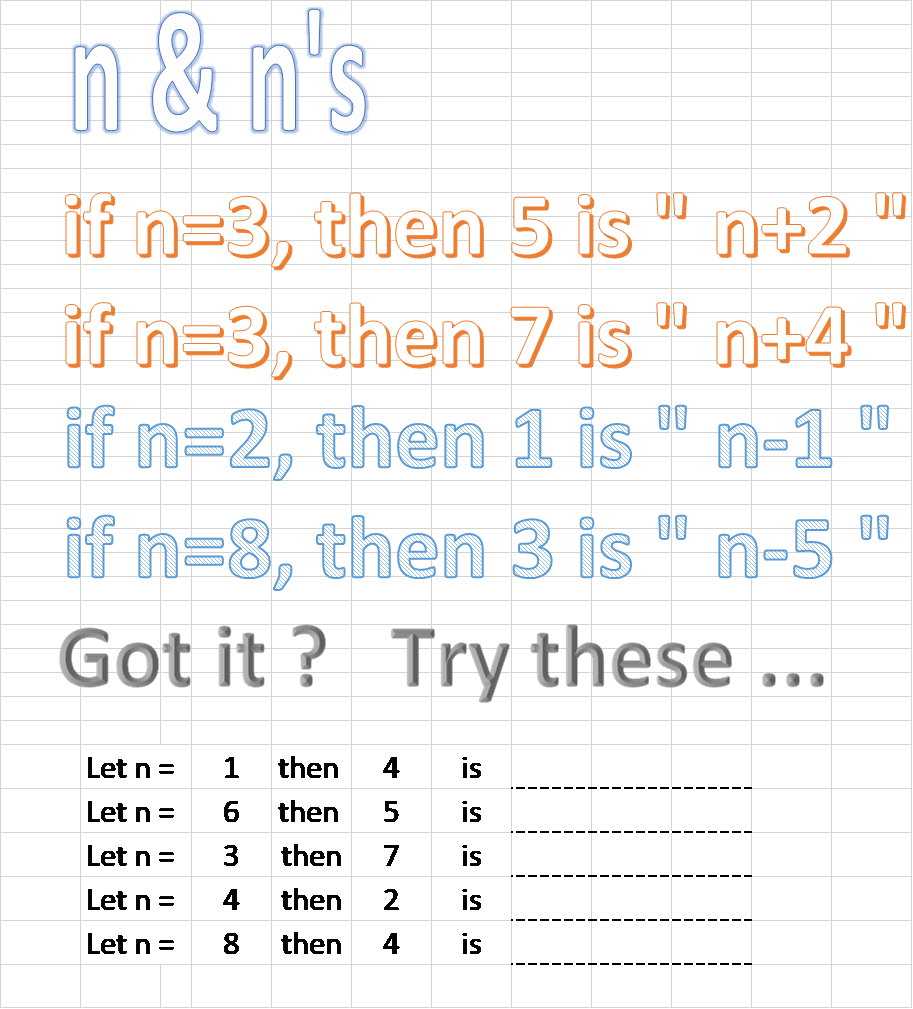
The ‘n&n’ task is undoubtably easy and sure enough it was for him but notice his use of ‘n-2’ and ‘n-4’. I prompted him for an alternative expression and he found ‘ n/2 ‘.

Part Three – moving from iconic to symbolic
For part three I asked my learner to explore change in a numerical context, so more symbolic than visual.
Question – Invariance & Change
What Stays the Same (invariance) and What Changes (change) for the three following expressions as a whole?
| 3 + 5 = 2 x 4, | |||
| 5 + 7 = 2 x 6, | |||
| 3 + 7 = 2 x 5 | |||
On writing the headings ‘Invariance’ and ‘Change’ he was seeing the language patterns and using them. His articulation was valid. He noticed operators ( x and +), and values (3+5=8, and 4 is half of 8) but he did not see the 4 as being in the centre of 3+5. Learners see different things.

Part Four – using the nth term towards a general formula
Only now in part four did my learner need to generalise. The idea here is that he has settled into ideas of ‘change’ and ‘invariance’ without having had an algebraic equation launched at him.
Question – invariance & change
Think back to the “ n & n’s challenge “, earlier. We are going to use this idea now.
The aim is to find a general expression for our three equations above.
- Take your first equation.
- Let n=3.
- Now follow through the remaining numbers, term by term, for each of those terms that you found earlier that change, and in so doing, develop a general expression.
Those terms that did not change, the ‘invariant’ terms, will just stay the same.
- Repeat, to obtain a general expression for the remaining two equations.
Test it – exploring the range of possible change for “ n “
We suspect that ‘ n ‘ is an integer, since we made it so when we first set it at 3, but now ask if your general formula works for other types of number.
Question
- Does it work for other whole numbers?
- Does it work for fractions?
- Does it work for square roots?
My learner’s generalising,
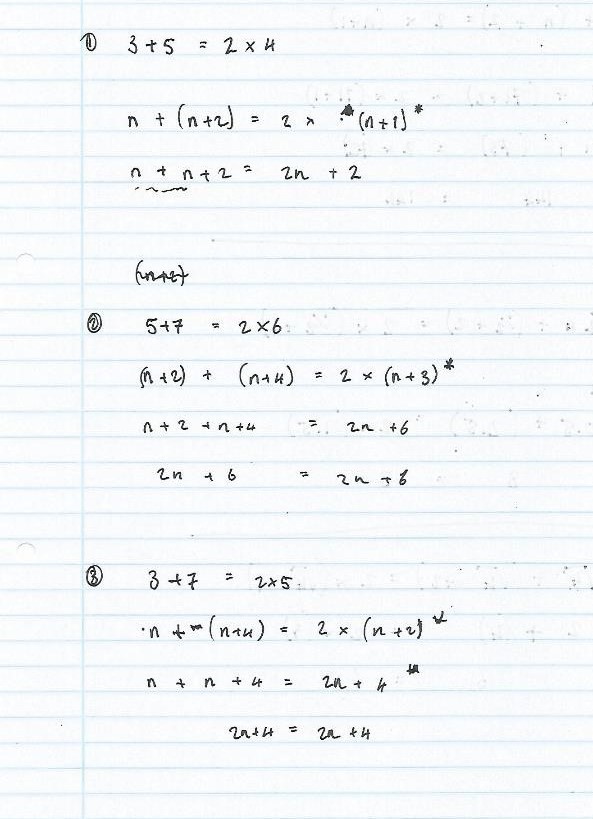
And his testing by specialising,
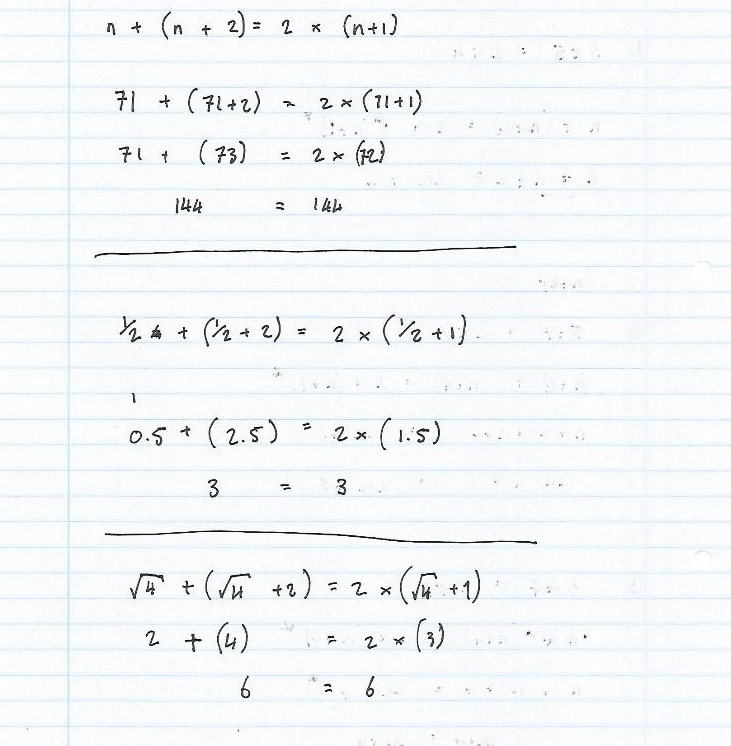
Part Five – can I create my own maths question?
School maths often ends there and the student thinks ‘so what?’ but now I encouraged my learner to push on and to create his own unique bit of maths using the theme of ‘Dimensions-of-possible-variation’.
Question – explore which bits of your general expression you can change
We want to create a whole new maths problem, which has the spirit of the existing, but which you alone have made.
- Looking now at your general expression, which elements can you change?
- Now change them.
- Make sure both sides of the equation still equate to each other
- Now insert some numbers to get three different expressions, much as the original question above did to start with.
This is your new maths problem. You could present these expressions to somebody and ask them “What Stays the Same and What Changes for these expressions as a whole?”
We reached a key learning moment; could he vary the generalisation? I did explain at this point what was being asked for (to adapt an element of your choosing in this general equation) but not what to vary. He hesitated, thought, and nervously said;
“The coefficient?”
I asked for more.
“n?” he said.
I told him to go ahead and our ‘n’ now morphed into 3n2
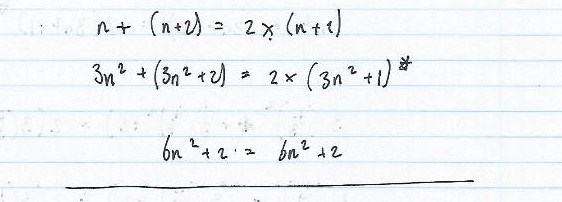
All that remained was to insert new numbers for ‘n’ and recreate the original question in his own vision. With care he substituted the numbers 2, and ⅓, and ¼ . Interestingly, despite squaring of fractions he did all of this without recourse to a calculator, which suggested competence.
Substituting for 2,
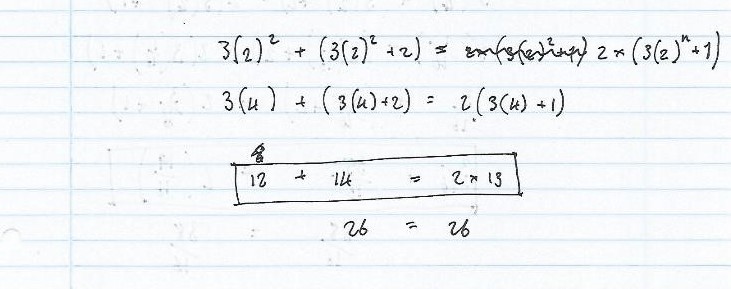
Substituting for ⅓, and ¼
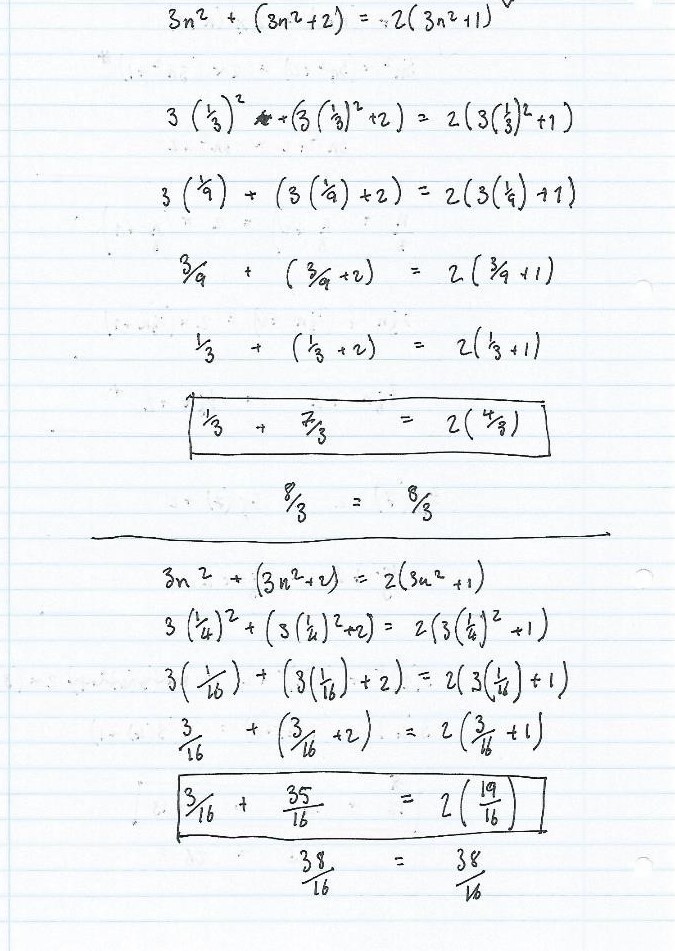

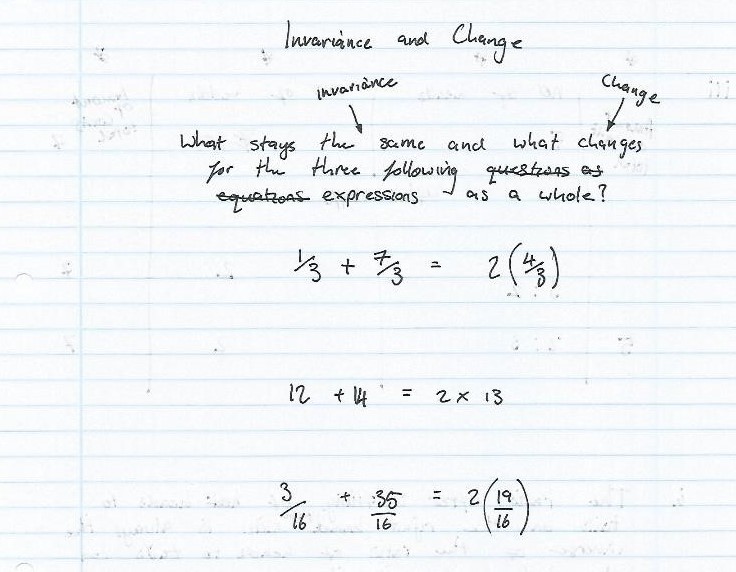
This was his unique version of the original question. He had used ‘Imagination’ and he had ‘Got a Sense of’ the themes of Invariance and Change.
His rewritten version of Task 13.1.5
Part Six – The Conclusion – is generalising relevant to me?
This part of the task involved no mathematical work and no question to do. It was given as a simple analogy. The idea was to appeal my learner’s Product Design skills and to explain and inform him as to what we mean by ‘developing thinking’.
Mathematical thinking, as shown in the above process, can be summarised:
- You are given a particular set of numbers in an equation.
- You generalise that equation, so it works for any number ‘ n ‘.
- You classify it as a simple linear relationship and explore its range.
- You manipulate it by changing those dimensions which can vary.
- You gain control over the structure of the problem.
- This control enables you to create a new and extended problem. This new problem is your brand.
Here is a simple analogy:
“You dedicate time to studying some particular timber materials; Ash, Birch, Ebony, Iroko and others. You generalise them into ‘Hardwoods’. You classify the hardwoods in terms of texture. You manipulate that texture into your proposed design of a piece of furniture. This gives you control over the look of the finished product. This control and finish gain you kudos. This kudos informs your brand.”
My reflections on the above task
I tried to conduct this question in the spirit of Jo Boaler’s piece on Sarah Flannery, European Young Scientist of the Year (Boaler, 2013). She writes (using her italics),
“The first thing I realized about learning mathematics was that there is a hell of a difference between, on the one hand, listening to maths being talked about by somebody else and thinking that you are understanding, and, on the other, thinking about maths and understanding it yourself and talking about it to someone else.”
I conversed with my learner on Task 13.1.5, explaining why we were doing the steps and what it achieved. He proceeded smoothly, using Invariance and Change in both spot-the-difference and in his generalising, but it seems my approach backfired somewhat. He was simply listening to ‘maths being talked about by me’. My conversing had failed in the chance to let him talk and reveal his own understanding. I then questioned why he had done so well and he said,
“You explained quite a lot, which made it easy.”
I immediately sensed didactic tension and realised that I could not now undo all of that talk. Did he just display behaviours that I had asked of him? Was he generalising or did he just blindly convert numbers into n’s? I sense a lot of the latter, though he did work actively on Dimensions-of-possible-variation, creating his own set of three equations, and likewise the work on Invariance and Change was all his own. Fortunately, the ‘Heads & Tails’ task came next, so I then felt the urge to do just the opposite and maintain a steady silence whilst he experienced the mathematical struggle, intervening only where needed.
Finding generality through a recreational maths problem
Posamentier’s problem on Heads and Tails
“You are seated at a table in a dark room. On the table, there are twelve pennies, five of which are heads up and seven of which are tails up. Now mix the coins and separate them into two piles of five and seven coins, respectively. Because you are in a dark room, you will not know if the coins you are touching were heads up or tails up. Then flip over the coins in the five-coin pile. When the lights are turned on there will be an equal number of heads in each of the two piles. How can this be possible?”
In ‘Heads & Tails’ I chose a question beyond the course material, and also as a way to challenge myself in applying the course techniques. I found the author’s original question (Posamentier, 2017) a little awkward (why sit in a dark room?) and he also revealed the solution, so in the spirit of the ME625 course textbook I developed it into a more eye-catching ‘Story Problem’, which related to my learner as a design student, and I kept the pattern hidden so I could register his surprise when he spotted the outcome of the puzzle, since if we could both take delight in his reaction then it acted as a motivator.

“You take a break from your studies, lean back in your chair, think about pizza, deadlines, assignments, software, formulae, design work, and you commit to acquiring a new set of watercolour markers for £11.99. You stare vacantly at the twelve pound-coins on your desk. The shops are shut. It’s late. Only the pizza place is trading.
You lean forward and playfully arrange five of the coins to form a group showing heads and the seven remaining coins to form a group showing tails. It looks concise, deliberate, and organised, but suddenly there’s a power cut. As the lights whine down you sit in the dark, waiting for something to reboot.
Nothing reboots!
The lights in the pizza parlour across the way have gone out too! Maybe it’s a temporary street-thing.
You think about reaching for your phone-torch but before you do you playfully shuffle the coins around in the pitch dark on your desk, and once again, by touch alone, separate them into a group of five, and a group of seven.
You flip over all the coins in the group of five.
The lights soon reboot, much to your delight, and you look down at the group of five and the group of seven coins, expecting something rather random.
Weirdly you notice something about the heads in each group!
How can that possibly be???”
Can you explain it;
i) in a sentence, that a friend or colleague could follow?
ii) in a diagram?
iii) using a table (ICT, not kitchen)?
iv) using a generalisation?
I had further adapted the task by asking the four questions shown above, three to help articulate, and a final challenging task of generalisation, with each step improving his understanding toward the next. The third question set up the problem on an Excel spreadsheet and my idea was to explore what awareness he had about the structure of the problem, which by this point should have been established. Would he see generalising or is it ‘just a spreadsheet’? At the final step he must generalise, but ‘finding x’ feels like ‘school algebra’. Would he still have absences in his grasp of it (you must first understand what ‘x’ is), despite progress on the earlier work?
The following photos show how the puzzle was played out after my learner had initially read the question and before he started on the written element.
This was the starting position, where heads were indicated by the white circles,

Now he shuffled the coins. We did not sit in the dark. I improvised a canopy to allow the coins to be hidden,

The coins are further shuffled prior to being flipped over, all still hidden under the canopy,

Joy was evident in this task. He whispered to himself upon reading the question, “So true …”. Idle time is universal. As the task unfolded for the very first time he said with sheer delight;
“No Way!! No way does that happen every time!”
He did it again and again. Same result!
Question (iii) – using a table
In approaching the questions my learner did not explicitly use the theme of ‘Do / Talk’ to himself or draft sentences nor even replay the pieces. Instead he sat quietly and thought and eventually drew the table shown below. He had unexpectedly opted to answer question three first, despite my spreadsheet-in-waiting. His table lacked good labelling to identify the flip of the coins but you can see that ‘3 and 2’ becomes ‘2 and 3’. It favours the iconic with its visual cues like columns and place-holders for his numbers. He had the number ‘2‘ at the foot of each column, which was the correct answer for that shuffle.

Question (i) – using a sentence
My learner moved onto question one. Articulating through a sentence is tricky. I could not make full sense of his and there was a struggle to clarify the ratio found in the table. Again, there was no reference to the flip of the coins, so it lacked detail.

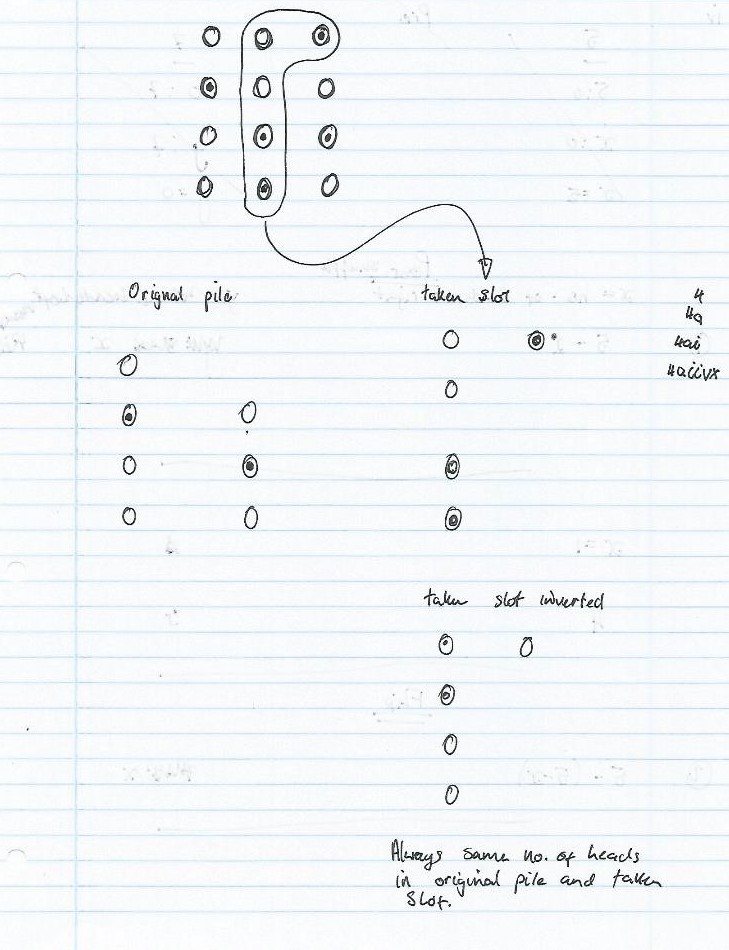
Question (ii) – using a diagram
Next he tackled question two but he was confused about a diagram. I had to first assist him on how to apply this diagram when moving from the enactive to the iconic. He followed my lead and then took a random selection of five circles, and then drew the flipped version, which allowed him to articulate the equal numbers of heads. You can see that his ‘Original Pile’ has two dotted circles and his ‘Taken Slot Inverted’ has the same.

Question (iii) – using my speadsheet
I now had to reintroduce question three with my pre-prepared spreadsheet. His task was to fill in the correct formulae in the cells. The Excel sheet is the key learning moment because inputting the cells is generalising and he recognised the need for the ‘Step-3 left cell’ to be a random number of heads (shown here as 32), and he succeeded with the formulae in Step-3 and Step-4 boxes with ease as they are simple subtractions or else unaltered. We did commence with 5 heads and 7 tails, but he soon experimented with 45 heads and tails.
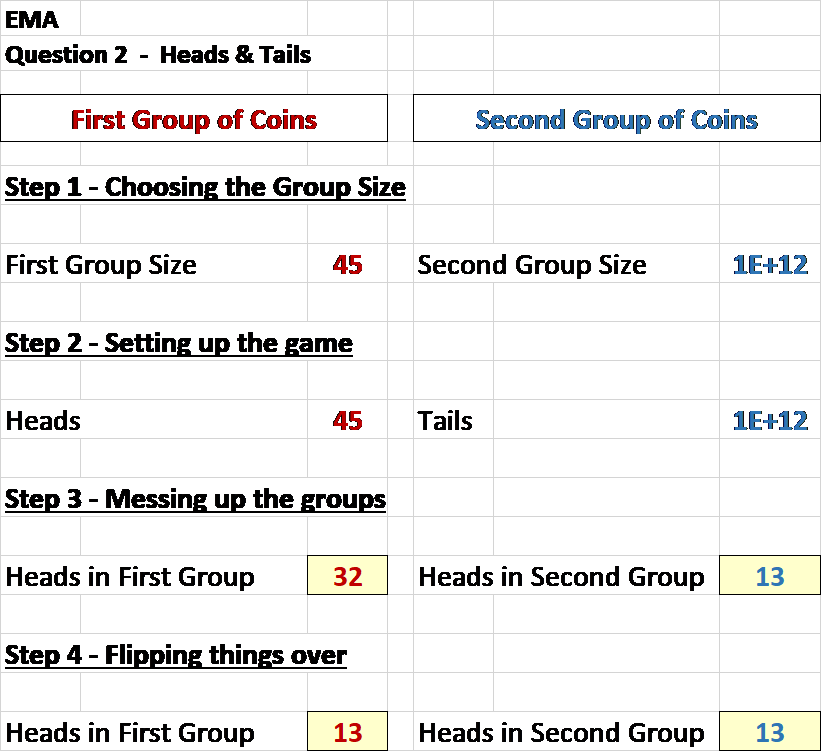
Question (iv) – using a generalisation
Question four proved the trickiest for my learner despite him now having some grasp of the situation from the previous three.
His generalising version began with two variables, x and y, but see how he ended up with y = x, which got a bit confusing and he then scribbled out the y variable. When the coins are flipped he wrote down ‘ 7-x ‘ at the foot of the page and then scribbled out the ‘ 7 – ‘. He was almost there.
His first attempt,

In his following page the algebra still seemed difficult to grasp for him. Here he has 1 and 6, so no matching heads. There was no sense of the theme ‘Do / Talk’ to himself first in an effort to grasp the unknown before attempting to solve. In the bottom of his page I had by now prompted him to “think of what x is”. See how he succeeded but only by clinging on to Specialising at the same time, here treating x as 1, (despite having two heads). It shows more confidence is needed.
His further attempt,
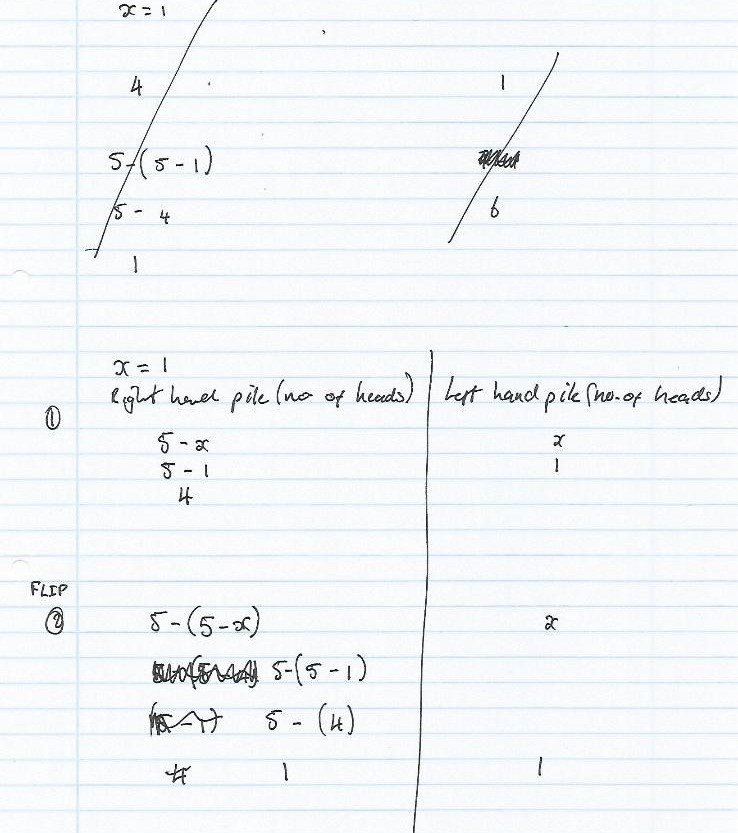
My Reflection on the above task
The ‘Heads & Tails’ task embraced one of Posamentier’s ‘Effective Techniques’ (Posamentier, 2016),
“Technique 4 – Entice the Class with a ‘Ghee-whiz’ Amazing Mathematical Result: One natural way to stimulate interest in mathematics among students is through the curiosity that nestles within all of us. Such curiosity can be awakened through new ideas, paradoxes, uncertainties, or complex results. Here the teacher’s talents come into play to find illustrations of easily understood situations that lead to unexpected results and leave the students intrigued (ghee-whiz), resulting in a motivation to pursue the topic further.”
Poems pare back word into some visceral cry and mathematical puzzles do likewise. This task offered that ghee-whiz result. My learner’s reaction, described above, resonated with this. It was sheer excitement at such a simple trick.
My learner Imagined-and-Expressed the process behind this trick in the form of a table. It was unexpected. I imagined he would sit for some time flipping and un-flipping coins (Do / Undo) to understand, but he thought it all through quietly in his mind. His table was accurate but see how his generalising fell apart in places. Where does the need for a second variable ‘y’ come from? It looked like a residue from linear equations (y=mx). No pause occurred to first grasp ‘x’ and follow its path from ‘5-x’ in one group, to ‘x’ in the other. It was hurried, evidenced by much scribbling. It was the last question-part so perhaps he was disengaging. Should I have intervened and talked more? It seems that conversing itself requires mastery. I asked him why, having found a general number in the spreadsheet, did he struggle with generalisation. Surely the spreadsheet simply ‘gave the game away’?
“It didn’t,” he said, “that was just filling in boxes, and besides those equations are all hidden anyway.”
So oddly, despite his setting up of those very generalised equations he could not easily connect that to generalising. Software, as powerful as it is, is not a panacea. It conceals. My view is that this is a problem of technique and method. He has not yet automated the concept of filling in Excel cells as a process of generality. More Do / Talk was required by him, both at the Excel and the generalising question. If I intervened at all it could have been to point this out. I was hoping to witness (maybe naively) the ‘Manipulate – Get a Sense of – Articulate’ spiral of increasing sophistication with each part. Instead it was curiously mixed.
Conclusion
Both tasks had the course textbook’s key ideas integral in their solutions and for me and my learner it showed that one can take ‘Developing Thinking in Algebra’ and apply it to the outside world, to problems randomly found in curiosity books. In other words, I could answer Root Questions using these general techniques.
Two things emerged from our solving:
- The level of verbal interaction in a lesson, and especially the learner talking of their maths understanding back to you, is extremely sensitive to how independently your learner will solve the problem.
- This conversation has to be tailored to your learner’s ability. This means that you have to know your learner (difficult in a large classroom).
It informed my approach to algebraic thinking, and serves my goal to seek control and power over the teaching process, and this is not dissimilar to the learner’s own quest for control and power over their maths problem.
Both draw inspiration from Kate Clanchy, on the poet Melissa Lee-Houghton, (Clanchy, 2019):
“She says, frankly and simply, that she suffers from depression and poetry is not the cure for it, but that poetry can give her a way of understanding and formulating herself, both as she writes it, and as she reads herself back afterwards. It gives her some distance and control.
The kids are mesmerized by this, and so am I. Control. Not turnaround but control. This word has somehow never occurred to me before, in all my anxious considerations of poetry and therapy, but it seems the right one.”
She adds,
“And if they dig deep, and find effective images, and make a good poem out of the truth of their lives, then that is not just control, but power. It’s different from being happy; it isn’t a cure for anything, but it is profoundly worth having. And actually, I don’t need anyone to tell me that; I know that from my own experience. I know it for myself.”
Care must be taken with such comparisons and the sense of control and power is by far an idiosyncratic thing but the spirit is the same; I want to understand, and dig-deep, and find effective methods, seek profundity, so as to be adept at producing the maths resource that converses, motivates and invokes the art of generalisation. Furthermore, what learner would refuse a chance at acquiring control and power; control in knowing how to start, where to start, preferred strategy, direction of travel and when to reverse, and of the power and delight when the problem yields because you fought for it, all of which alleviates (not cures) your maths fear.
Interestingly, none of it is for turnaround of the student, and the course textbook itself states, arguably, “I cannot change others”. The end desire is that the learner will change of their own volition, much as I have done in the course of my own algebraic thinking.
Seeking such personal power and control is rooted in human nature. It is enlightening to see ‘Developing Thinking in Algebra’ tie mathematical power to the humanist subjects of English and History, and by the prompting of learners to “develop their powers to imagine, and to express what they imagine to others”. We can rejoice that this notion is still vibrant thirty-three years after Davis & Hersh (Davis & Hersh, 1986) embraced it when the omnipresent I.C.T. was still cutting its coding teeth,
“Metaphor and analogy exist in mathematics and physics as well as in poetry and in religion. Rhetoric exists in mathematics (despite claims to the contrary) as it exists in politics. Aesthetic judgement exists in mathematics as it exists in the graphical or performing arts.”
Those authors wholly reclaim mathematics as being a human institution, and if it ever ceases to be so then they argue that we must “let it decay”.
References
Boaler, Jo. 2013. The Elephant in the Classroom. Souvenir Press.
Clanchy, Kate. 2019. Some Kids I Taught and What They Taught Me. Picador.
Davis, Philip. Hersh, Reuben. 1986. Descartes Dream. Penguin Group.
The authors conclude on page 305, “If a synthesis cannot be achieved, if it comes to a showdown between man and mathematical science, then man would be best advised to stop the process. Let it fall into decay…”
Mason, John. Graham, Alan. Johnston-Wilder, Sue. 2012. Developing Thinking in Algebra. The Open University in association with Sage Publications Ltd.
Posamentier, Alfred S. Krulik, Stephen. 2016. Effective Techniques to Motivate Mathematics Instruction. Routledge.
Posamentier, Alfred S. 2017. The Joy of Mathematics. Prometheus Books.
