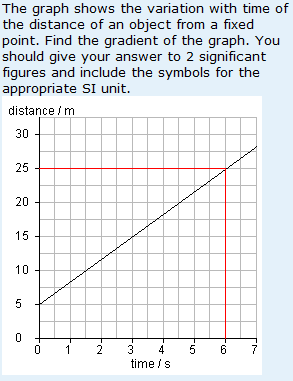
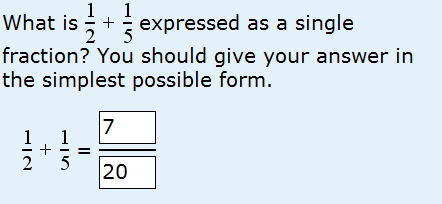
I’ve talked about students’ difficulties with units before – on 10th and 15th March 2011. In addition to the deeper problems that students encounter, they frequently give the incorrect case when writing abbreviations for units. When they write Kg instead of kg for kilograms in an answer to an iCMA question, I have a certain amount of sympathy (especially since K and k are completely impossible to distinguish in many people’s handwriting – including mine!). However Kg is just plain wrong, so I would be unhappy to give full credit for an answer that included this error. It is really important to give targeted feedback on errors of this type.
Students also get the case wrong when the correct case of a unit is more obvious e.g. they write j instead of J for joules. And they make similar errors in writing the case 0f variables e.g. in answer to a high-stakes question where the correct answer is nRT, more than 1% of responses were only incorret because of case, with many responses of nrt, NRT etc. I find it slightly disappointing that students don’t seem to appreciate that things like this matter (even though we have explained this in the teaching text).